Transverse Wave

1. Crest

The crest is the highest point or “peak” reached by the wave particles above their equilibrium position.

2. Trough

The trough is the lowest point or “valley” below the equilibrium position. It’s the opposite of the crest.

3. Wavelength

The wavelength is the distance between two consecutive crests or troughs. It is usually measured in meters and determines how “long” a wave is.

4. Amplitude

The amplitude is the maximum displacement of particles from their equilibrium position, either above or below it. It indicates the wave’s energy: larger amplitudes mean more energy is carried by the wave.

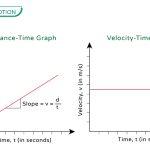
5. Frequency and Period

The frequency is the number of complete waves that pass a point in one second, measured in hertz. The period is the time taken for one complete wave cycle to pass a point, given by the reciprocal of frequency:

\[ T=\frac{1}{f} \]

1. Water Waves

Water waves are a classic example of transverse waves. On the surface, water particles move mostly up and down, perpendicular to the wave’s horizontal direction of travel. However, they also have a slight circular motion.

2. Light Waves

Light waves are electromagnetic waves. They consist of oscillating electric and magnetic fields perpendicular to each other and the direction of wave propagation. Unlike water waves, light waves do not require a medium and can travel through a vacuum.

3. Guitar String

When a string on a guitar is plucked, transverse waves are generated. The string moves up and down while the wave travels along its length. These vibrations can produce standing waves, where specific points (nodes) remain stationary while others (antinodes) oscillate with maximum amplitude.

The wave equation is a fundamental relationship that connects a wave’s speed, frequency, and wavelength. It helps in analyzing how waves propagate through different media and is expressed as:

\[ v = f \lambda \]

Where:

– v: Wave speed (ms^-1 )

– f: Frequency (Hz)

– λ: Wavelength (m)

This equation illustrates that the speed of a wave is directly proportional to its frequency and wavelength.