Momentum
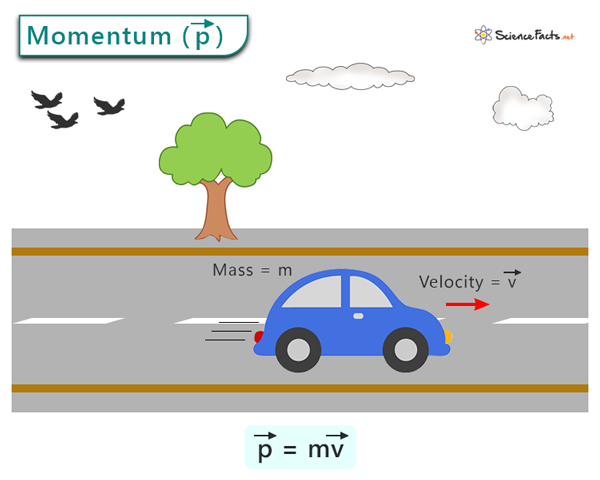
Momentum is a fundamental property in physics and is defined as the product of the object’s mass and velocity. It is a vector quantity having both magnitude and direction. A car moving on a road has momentum. A soccer ball gathers momentum when it is kicked.
Formula
Since momentum is the product of mass and velocity, it is given by
Momentum = mass x velocity
p = mˑv
Where
p is the momentum
m is the mass
v is the velocity
In vectorial notation
The momentum along the positive axis direction is considered positive, while the momentum along the negative axis direction is considered negative.
Unit & Dimensions
The SI unit for momentum is kgˑm/s. The cgs unit is gˑcm/s. Its dimensional formula is [M1 L1 T-1].
Momentum is a quantity involving the motion of an object. Objects with greater mass naturally have high momentum, but this will also depend on velocity. Suppose a car and a truck move on the highway with the same velocity. Both have momentum. The truck is heavier than the car, so it will have higher momentum.
Change in Momentum
Change in momentum is a useful quantity, as we shall explore later. Suppose a moving object changes its velocity (Δv) at any time. It experiences momentum change. The change in momentum (Δp) is the difference between the final and initial momentum values.
Δp = mvfinal – mvinitial
=> Δp = m(vfinal – vinitial)
=> Δp ꓿ mΔv
Momentum, Force, and Impulse
From Newton’s second law of motion, the force is given by the product of mass and acceleration. Newton also stated his second law of motion in terms of momentum: The net external force equals the change in momentum of a system divided by the time taken for the change to occur.
Fnet = Δp/Δt
=> Δp = Fnet x Δt
The term Fnet x Δt is known as an impulse. This equation shows that the impulse equals the average net external force multiplied by the time the force acts. It measures how quickly force changes in magnitude and direction. It is also equal to the change in momentum. The effect of a force on an object depends on how long it acts, as well as the strength of the force.
Impulse is a valuable concept because it quantifies the effect of a force. A vast force acting for a short time can significantly affect an object’s momentum, such as the force of a racket hitting a tennis ball. A small force could cause the exact change in momentum, but it would have to act much longer.
Application
An application of the momentum equation is to study collision between objects. Momentum is a conserved quantity. When a collision occurs, the total momentum before the collision equals the total momentum after the collision. It is known as the law of conservation of momentum and is explored in another article.
Example Problems
Problem 1. A ball of mass 0.7 kg is thrown with a velocity of 15 m/s. What is the momentum of the ball?
Solution
Given m ꓿ 0.7 kg and m ꓿ 15 m/s
The momentum is
p ꓿ mv ꓿ 0.7 kg x 15 m/s ꓿ 10.5 kg·m/s
Problem 2. A car of mass 980 kg travels at a speed of 45 m/s when it slams on the brakes and stops in 7 seconds. What is the momentum 3 seconds after it slams the brake?
Solution
Given m ꓿ 980 kg, v ꓿ 45 m/s, t = 7 s
The acceleration (a) can be obtained from the following equation.
v = u – at
=> 0 = 45 m/s – a x 7 s
=> a = 6.43 m/s2
Velocity after 3 seconds is
v = u – at = 45 m/s – 6.43 m/s2 x 3 s = 25.7 m/s
The momentum is given by
p ꓿ mv
=> p ꓿ 980 kg x 25.7 m/s ꓿ 25,200 kg·m/s
Problem 3. (a) Calculate the momentum of a 105 kg football player running at 9 m/s. (b) Compare the player’s momentum with a 0.390 kg football moving at 30 m/s.
Solution
The momentum is given by
p ꓿ mv
(a) The momentum of the football player is
p ꓿ 105 kg x 9 m/s ꓿ 945 kg·m/s
(b) The momentum of the football is
p ꓿ 0.390 kg x 30 m/s ꓿ 11.7 kg·m/s
Therefore, the player’s momentum is 80 times more than the ball.
Although the ball has a greater velocity than the player, its momentum is low because of its low mass. When the player catches the ball, there will not be much impact on his momentum.
Problem 4. 2. A car has 18,000 units of momentum. What will be its new momentum if
a. its velocity is doubled?
b. its mass is doubled (by adding more passengers)?
c. both its velocity and mass doubled?
Solution
Given
p ꓿ 18,000 units
The momentum is given by
p ꓿ mv
a. If the mass is doubled, the new momentum is
pnew ꓿ 2m x v ꓿ 2 x 18,000 ꓿ 36,000 units
b. If the velocity is doubled, the new momentum is
pnew ꓿ m x 2v ꓿ 2 x mv ꓿ 2 x 18,000 ꓿ 36,000 units
Therefore, the momentum doubled when either the mass or the velocity doubled.
c. If both the mass and the velocity are doubled, the new momentum is
pnew ꓿ 2m x 2v ꓿ 4 x mv ꓿ 4 x 18,000 ꓿ 72,000 units
Therefore, the momentum quadrupled when both the momentum and velocity doubled.
-
References
Article was last reviewed on Friday, July 28, 2023