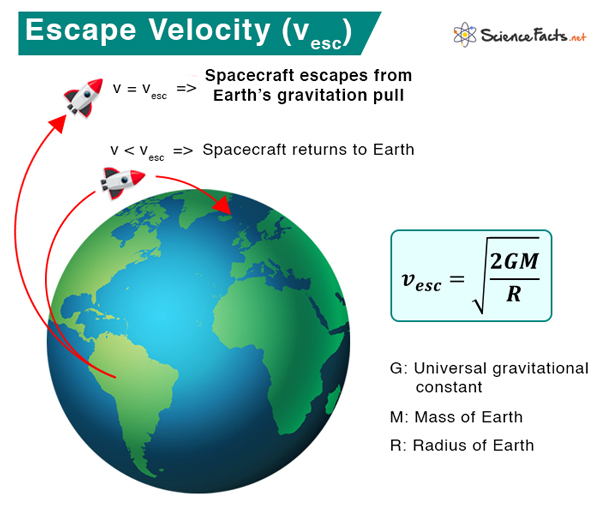
Escape Velocity
If you throw an object straight up, it will rise until gravity stops it and returns it to Earth. The force of gravity reduces as the distance from the center of the Earth increases. However, suppose you can throw the object with enough initial upward velocity so that decreasing force of gravity does not completely slow it to a complete stop. In that case, the velocity can always be high enough to overcome the Earth’s gravitational force. The initial velocity required to attain that situation is called escape velocity.
Escape velocity is the speed required for an object to be projected to overcome the Earth’s gravitational force. The object escapes from Earth’s surface into space without ever falling back.
Formula
The equation for escape velocity is as follows:
Where
vesc is the escape velocity
G is the universal gravitational constant (= 6.67 x 10-11 m3 ˑ kg-1 ˑ s-1)
M is the mass of the earth (= 5.972 x 1024 kg)
R is the radius of the earth (= 6.371 x 106 m)
Escape Velocity of Earth
The escape velocity on Earth’s surface is
Therefore, the minimum velocity required to project an object from Earth’s surface to escape the gravitational pull is 11.182 km/s or 25,000 mph. Scientists use this value to send rockets into space.
Example Problem
If the mass of Mars is 6.41 x 1023 kg and its radius is 3.39 x 106 m, what is the escape velocity of an object launched from Mars?
Solution
Given
MMars = 6.41 x 1023 kg
RMars = 3.39 x 106 m
G = 6.67 x 10-11 m3 ˑ kg-1 ˑ s-1
The escape velocity from Mars is
The following table lists the escape velocities of several planets, the sun, and the moon.
| Planetary Body | Escape Velocity (km/s) |
|---|---|
| Earth | 11.2 |
| Mars | 5.02 |
| Jupiter | 59.5 |
| Mercury | 4.3 |
| Venus | 10.36 |
| Pluto | 1.21 |
| Sun | 617 |
| Moon | 2.38 |
Derivation
We use the energy conservation principle to derive the expression for escape velocity. The kinetic energy of the object is
Where m is the object’s mass and v is its velocity.
The gravitational potential energy on the surface of the earth is
From the law of conservation of energy, the kinetic energy comes from the gravitational potential energy.
In the escape velocity calculation, we assume no friction with the Earth’s atmosphere.
After achieving the escape velocity, the object does not require any impulse to escape from Earth’s gravitational field. The object achieves zero velocity asymptotically at an infinite distance from the Earth’s surface, never to return.
-
References
Article was last reviewed on Friday, July 28, 2023