Angular Displacement
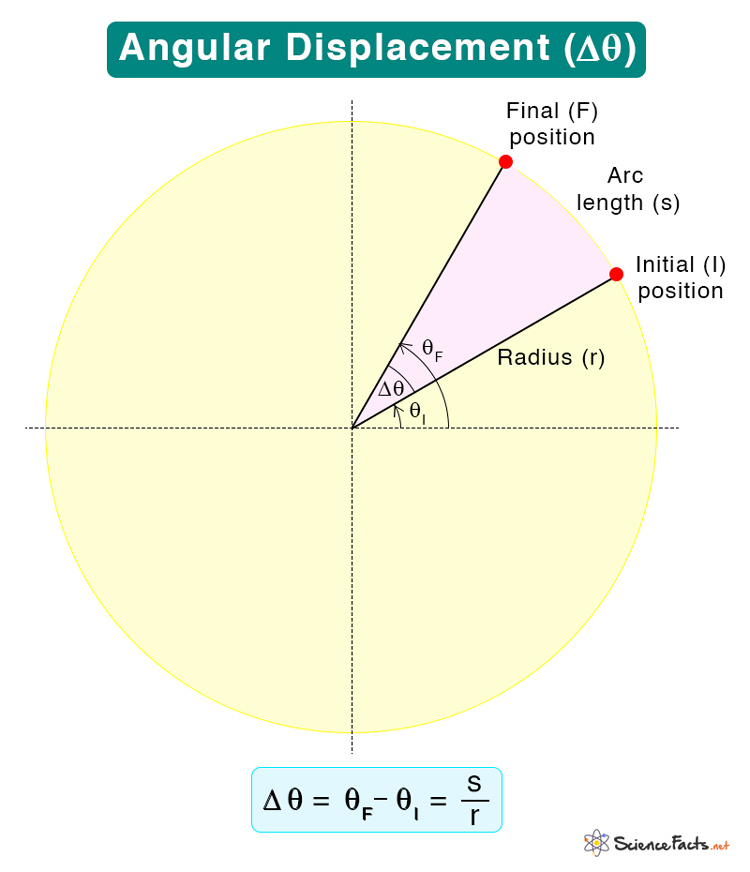
A circular or curvilinear motion describes the motion of an object in a curve path. When the object moves along a curve path from one position to another, the angular displacement quantifies how much it has moved. It is the angle by which the object is displaced, as shown in the image below.
Angular displacement is analogous to linear displacement. However, during linear displacement, the object moves in a linear path. On the other hand, during angular displacement, the object moves in a circular path.
Angular Displacement Equation
An object moving along a circular path sweeps out an angle about its axis of rotation. One can measure the change in this angle to the object’s initial position. Imagine a line joining the object from the axis of rotation. As the object sweeps an arc, the line rotates. The angular displacement is the angle between the rotating line’s final and initial positions.
Δθ = θf – θi
Where
Δθ: Angular displacement
θf: Final position of the object
θi: Initial position of the object
Symbol: Δθ (delta theta) or θ
Unit: Degree or radian (1 radian = 57.3 degrees)
Angular Displacement From Linear Displacement
There is another way to calculate the angular displacement. Suppose s is the arc length and r is the object’s distance from its axis of rotation. r is also the radius of the circular path the object makes. The angular displacement is
Angular displacement = arc length/radius of the circular path
θ = s/r
The arc length is almost equal to the linear displacement. Therefore, the above equation establishes a relationship between linear and angular displacements. It can be seen that the two are proportional to each other. If the linear displacement increases or decreases, the angular displacement will also increase or decrease.
On the other hand, the angular displacement is inversely proportional to the radius. Suppose two objects with different radial distances rotate about a common axis with the same angular displacement. The object further from the axis will traverse a longer linear distance than the one closer.
Angular Displacement, Angular Velocity, and Angular Acceleration
The rate of change in angular displacement is called angular velocity. The rate of change of angular velocity is called angular acceleration. The angular displacement (θ), angular velocity (ω), and angular acceleration (α) are related by the following equation.
θ = ωt + ½ αt2
Example Problems
Problem 1: A person walks around a circular track of diameter 9.5 m. If he walks for a distance of 70 m, what is his angular displacement?
Solution
Given,
diameter (d) = 9.5 m => r = d/2 = 9.5 m/2 = 4.75 m
linear displacement (s) = 70 m
The angular displacement (θ) is given by
θ = s/r
or, θ = 70 m/4.75 m = 14.7 radians
Problem 2: Find the linear distance traveled by an object on a circular path of radius 8.25 m with an angular displacement of 0.358 radians.
Solution
Given
radius (r) = 8.25 m
angular displacement (θ) = 0.358 radians
The linear distance (s) is given by
s = rθ = 8.25 m x 0.385 radian = 3.18 m
-
References
Article was last reviewed on Monday, January 2, 2023